It is said that old pump men never die—they just lose their prime—and I fear that is partially true. Therefore, before I lose my prime, I thought I'd share some equations with some of you younger pump-heads, which allow you to calculate the best efficiency point (BEP) capacity and head of a centrifugal pump, knowing only certain impeller and casing dimensions and the pump rotative speed. I have found it quite useful to be able to predict the hydraulic characteristics of a centrifugal pump before it is built, even before the drawings are made. I've also found it useful to be able to predict the effect of changing any of the pump design parameters, even using an impeller from a different pump.
For years, engineers used constants Km1 and Km2 from Stepanoff's Figure 5.21 to design impellers. The results were generally unsatisfactory. As a replacement for this procedure, I derived the equations presented in this column and have found them to be pretty accurate for pumps with radial-flow impellers, volute casings (single and double) and specific speeds from 500 to 2,500 (U.S. units). You will note many references to Walter Jekat's milestone document2, because he provided all the pieces to the puzzle, while I simply condensed and assembled them to produce these equations.
The Equations
The impeller and casing dimensions required for the calculations are shown in Figures 1 and 2.
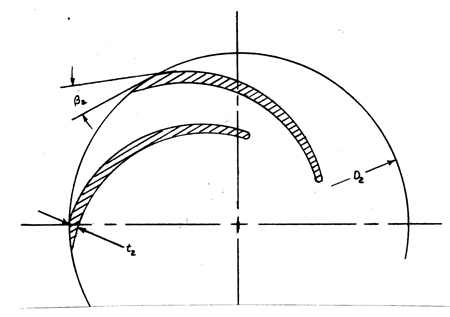
Figure 1. Partial impeller plan view showing equation performance parameters
Equation 1 predicts the BEP capacity.
Where:
Qbep = Pump flow rate (capacity) at BEP (U.S. gal/min)
u2 = Velocity of the impeller outside diameter (ft/sec) = D2N/229
N = Rotative speed of impeller (rev/min)
R4 = Distance from the center of the shaft to the center of the casing throat (inches)
A4 = Area of the casing throat (square inches)
D2 = Outside diameter of the impeller (inches)
µ = Slip factor—the ratio of the tangential components of the actual absolute impeller discharge velocity to the “theoretical” absolute impeller discharge velocity
C = Correction factor to allow for the boundary layer in the casing throat
From Jekat2:
= 1.0 for volutes of large pumps
= 0.9 for commercial, cast volutes of small and medium size pumps
= 0.8 for vaned diffusers
ß2 = Angle between the tangent to the impeller outside diameter (OD) and the tangent to the impeller vane at the impeller outlet (D2) (degrees)
ηv = Volumetric efficiency, as a decimal, typically 0.97. A more precise estimate can be obtained from Jekat2.
AR2 = Total impeller exit flow area, between the vanes, measured normal to the liquid flow (square inches)
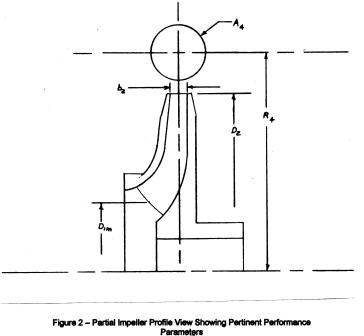
Figure 2. Impeller profile view showing equation performance parameters
The best equation I've found for the slip factor, µ, is Equation 2, provided by Jekat2, which he attributes to Pfleiderer.
Where:
a = Casing configuration factor. From Jekat2: for volutes - 0.65 to 0.85; for vaned diffusers – 0.6; for vaneless diffusers – 0.85 to 1.0
Z = Number of impeller vanes at the OD of the impeller
D1m = Diameter of the midpoint of the impeller vane on the pressure side at the inlet (inches)
AR2 can be approximated with Equation 3.
AR2 = b2(πD2tanß2-Zt2)
Where:
b2 = Width of the impeller vane at the OD of the impeller (inches)
t2 = Thickness of a vane at the OD of the impeller, measured normal to the vane surface (inches)
Equation 4 is the best equation that I've been able to produce for predicting the head at BEP. It assumes negligible pre-rotation of the fluid entering the eye.
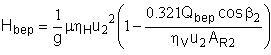
Where:
Hbep = Head produced by the pump at BEP (feet)
g = Gravitational constant (32.2 ft/sec2)
ηH = Hydraulic efficiency, as a decimal
Equation 5 from Jekat2 approximates the hydraulic efficiency.
ηH = 1- 0.8/Q0.25
Example
The following dimensions were measured from a horizontal, 4 x 6 multistage pump with a twin-volute casing:
Z = 5 vanes in each impeller
ß2 = 23 degrees
D1m = 4.2 inches
D2 = 10.9 inches
b2 = 0.5 inches
t2 = 0.2 inches
R4 = 6.3 inches
A4 = 3.6 square inches (both volutes)
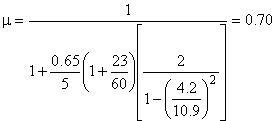
u2 = D2N/229 = (10.9)(3550)/229 = 169 feet/sec
AR2 = 0.5(π(0.9)tan230 – (5)(0.2)) = 6.8 square inches
= 812 gallons per minute
ηH = 1 – 0.8/8120.25 = 0.85
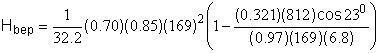
= 414 feet
The published curve for this pump showed a Qbep of 850 gallons per minute and an Hbep of 383 feet per stage. The calculated Qbep is only about 4 percent low, which is pretty close considering all the approximated values used in our calculations and the rounding of the measured dimensions. The calculated head is about 8 percent high. An oversize impeller eye could account for the capacity being higher than calculated and the head being lower than calculated (because of pre-rotation). However, the evaluation of the eye indicated that it was appropriately sized. An examination of the pump casing revealed the probable causes of the unnecessary head losses in the return passages, which may account for at least part of the 8 percent deficiency in the pump head.
P&S
References
1. Stepanoff, A. J., Centrifugal and Axial Flow Pumps, John Wiley & Sons, New York, N.Y., 1948.
2. Jekat, Walter K., “Centrifugal Pump Theory”, Section 2.1 of the first edition of the Pump Handbook, edited by Karassik, Krutzsch, and Fraser, McGraw-Hill Book Co., New York, N.Y., 1976.
Pumps & Systems, May 2011