by Lev Nelik
07/23/2012

For a quick estimate of pump head in the field, the discharge pressure gauge is read. This number is multiplied by 2.31, and operators can get a rough value of the pump head. If the gauge reading is 80 psig gauge, then:

Head = 80 x 2.31 = 185 feet

Knowing the head and having a performance curve available, you can read back from the curve and have  the flow. Many times that is a prime objective—to know, albeit approximately, the flow rate. Therefore, this method is acceptable.

However, while quick and simple, this method ignores many variables that could distort the answer. Some of these distortions can be insignificant, and others may greatly affect the number.

 

The Basics

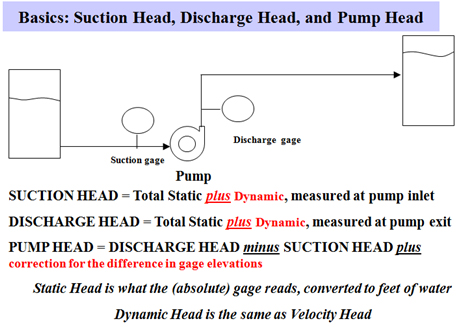
Consider the basics: pump head is the difference between the total energies of the discharge side and suction side. This total energy has three components:
•    Static head —a difference between the readings of the discharge and suction gauges, expressed in feet
•    Velocity head difference
•    Elevation difference—between readings of the discharge and suction gauges

 

A More Accurate Method

What would the results be if we attempt to compute this more accurately? How far would we be from the quick value of 185 feet of head?
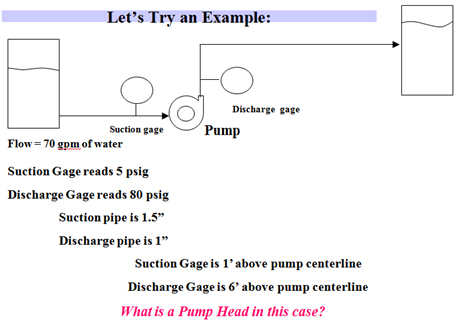
Let’s look at another example. A pump moves 70 gallons per minute (gpm) of water, and the suction gauge reads 5 psig, and the discharge gauge reads 80 psig. The piping connected to the pump is 1.5 inches at the suction and 1 inch at the discharge. The suction gauge is 1 foot above the pump’s centerline. The discharge gauge is 6 feet above the pump centerline. What is the pump head with these numbers?

Note: Can you confirm—for free registration to the next Pump School session—that 0.321 is a correct conversion constant to make the units come out correct?

 

 

 

 

 

At the discharge, the area and velocity are:

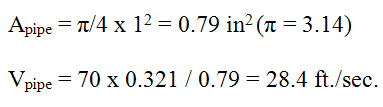
At the suction, the area and velocity are:

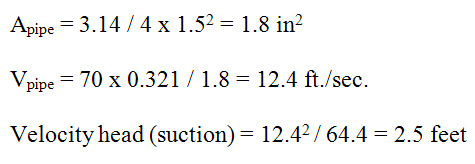
To get everything in consistent units (accounting for atmospheric pressure), in absolute units:

We came amazingly close—185 feet using the quick method versus 188 feet using the more accurate method—accounting for all the factors that can affect the calculation and spending extra time on the calculations). Does this mean that correcting for velocity head and gauge elevation is a waste of time? The answer to this question will be answered in the second part of this series in the September issue.

 

Readers Respond

If you use a modification of Bernoulli’s Equation in which you include the pump as a component and ignore head loss as it is accounted for in the gauge readings, the answer is readily solved, including corrections for gauge locations with respect to the pump centerline.  
 
By the way, shouldn’t suction head include the 1 foot for the gauge location above the centerline? If you were to move the suction gauge to the centerline of the pump, the gauge reading would be higher by 1 foot. 
 
I have done this many times when I have had a long flexible pressure hose attached to the gauge. I have moved the gauge up and down and watched the pressure reading on the gauge rise and falls accordingly. Likewise, for the discharge head, the pump is going to see 6 feet of head to the point where the gauge is attached, even if the gauge does not read it.
Chuck Anderson
Strand Associates, Inc.
 
Lev Nelik responds: 
Chuck & Lyn (see Lyn’s answer on page 5), thank you for your nice work going through the “assignment” from the August column. Both of your inputs are related, and I am combining my response to you in one.
 
You both did great deriving the popular 0.321 conversion coefficient between flow and area. Also, regarding the gauges, to Chuck’s point, elevations can be added to both suction and discharge head (after which the pump head would handle that as a difference. The corrected (6 – 1 = 5) feet can be added to the pump head, which is first calculated accounting for pressure and velocity only.
 
Lyn, if I am not mistaken, you have contributed input to my earlier articles? It sounds familiar, but I cannot recall the topic.
 
Image of Chuck Anderson's answer
Chuck Anderson’s answer
 
Chuck Anderson responds:
My comment was that to get a true total suction head and total discharge head, the correction for gauge location should be included in each, and the difference works out to the pump head.
 
Also, I did comment on an NPSH article that you wrote in, I believe, April of 2011.
 
Lev Nelik responds:
Exactly! Great point. Thanks, Chuck.
 
Lyn Greehill answers:
This is my derivation of the conversion factor in your article on velocity head.
 
Calculation of Flow Velocity from Flow Rate and Area
The equation to convert flow rate and area into velocity is:
 
 
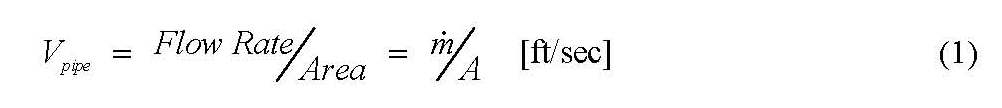Equation 1
 
Given the flow rate in gallons per minute (gpm) and the area in square inches (in.2), what is the appropriate conversion factor to apply in Equation 1 to obtain pipe velocity in units of feet per second? In equation form, the conversion can be written:
 
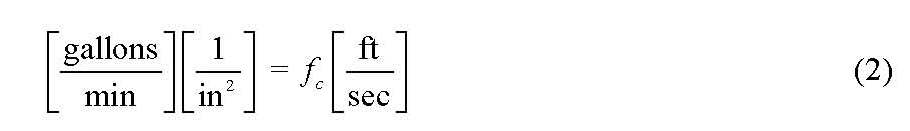Equation 2
 
where fc in Equation 2 is the desired conversion factor. Noting that in the U.S., 1 ft.3 is 7.48 gallons of liquid, the following multipliers are used to determine the conversion factor:
 
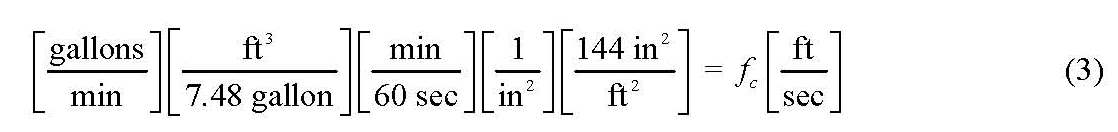Equation 3
 
Multiplying the conversions in Equation 3, the desired factor is:
 
Equation 4
 
As such, to convert flow in gpm and pipe area in square inches to velocity in feet per second, expressed in Equation 2, divide the pipe area into the flow and multiply by the 0.321 conversion factor as calculated in Equation 4.
Lyn Greenhill, PE
DynaTech Engineering, Inc.


Read part 2 of this story here.

 



Dr. Nelik (aka "Dr. Pump") is president of Pumping Machinery, LLC, an Atlanta-based firm specializing in pump consulting, training, equipment troubleshooting and pump repairs. Dr. Nelik has 30 years of experience in pumps and pumping equipment. For more information, visit www.pump-magazine.com.