Power factor (PF) is an important component of an alternating current (AC) circuit, but understanding its actual effect can be difficult. Why is PF mysterious to many of us? It has to do with the way it is explained. PF is often defined as the ratio of the real load power to the apparent power in the circuit. Real power is the power consumed by the circuit, and apparent power is the sum of the real power and reactive power. Reactive power is often called imaginary, phantom or borrowed power. Real power is measured in watts, and reactive power is measured in volt-amps. Isn’t a watt a volt-amp? Maybe not, if those amps are imaginary. Another explanation uses a vector triangle to show the relationship of real, reactive and total power. This can be useful to some, but confuses others. Most simply, PF can be defined as the cosine of the phase angle between the voltage and apparent power. When I presented my AC Power and Motors seminar in the late 1990s, I used some of the information above to explain power factor. The result was that some of the audience gained an understanding, but the majority did not. In 2007, I changed how I presented the topic. Today, the majority say that they understand it, but a small minority still do not. Although the results were not perfect, they are better than they used to be. I will use my current approach in this column. Although several inductive devices contribute to PF, this column will discuss AC motors. Rather than power, the rise and fall of voltage and current during the AC cycle will be the focus. The illustrations are from my current AC power and motors seminars.
What Is Power Factor?
PF indicates how effectively an AC motor uses current to perform work (torque) and ranges from 0 to 100 (0 to 1). For example, assume that a 1-horsepower, 230-volt, single-phase motor is 100 percent efficient. If the motor power factor is 100 (1), which is impossible, the utility would have to supply just 3.24 amps (746 watts) for the motor to run at full load. At a more realistic power factor of 75 (0.75), the utility would have to supply 4.32 amps (994 watts). Although the additional amperage is not consumed by the motor, the utility still must provide it or the motor could not operate at full load. Successful motor operation is “a tale of two currents,” in that two types of current are required: the load current, which performs work; and the magnetizing current, which initiates a magnetic field in the stator. Charles Dickens might have summed it up this way: “It was the best of currents, it was the worst of currents.” The motor uses load current to do work. The load current increases or decreases based on the actual load on the motor. It is never zero, even when disconnected from the load, because of the friction and windage associated with the rotor and bearings. Figure 1 shows one complete AC cycle (360 degrees). The blue curve is voltage, and the other three curves are the load currents at various motor loads. The current at all loads rises and falls synchronously (in phase) with voltage and reaches its peak values at 90 and 270 degrees. At any point during the cycle, the power in watts is equal to the product of volts and amps because it is a true resistive load.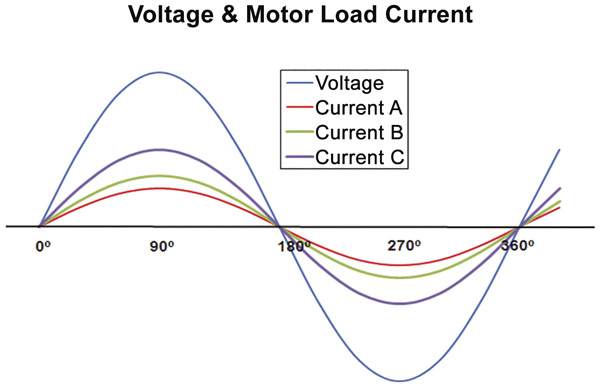 Figure 1. Load current versus voltage (Graphics courtesy of the author)
Figure 1. Load current versus voltage (Graphics courtesy of the author) Figure 2. Magnetizing current versus voltage
Figure 2. Magnetizing current versus voltageHow Is Power Factor Calculated?
Figures 1 and 2 illustrated how load and magnetizing currents rose and fell during one cycle of AC. Load current was in phase with voltage, but magnetizing current lagged voltage by 90 degrees. Figure 3 shows the effect of both during one cycle. The red curve is load current, and the green one is magnetizing current. In this example, I chose to show both with the same current intensity to keep the results simple. In the case of a fully loaded motor, the load current will be substantially higher than the magnetizing current.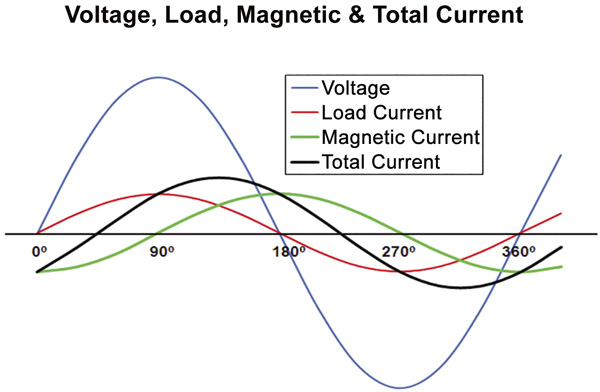 Figure 3. Total current versus voltage
Figure 3. Total current versus voltage
