How do you calculate the leakage rate of a mechanical seal?
Mechanical seals are precision devices that are designed to seal a rotating shaft; however, they do rely on a small amount of leakage to lubricate and cool the seal faces. Leakage provides the lubricating film, which is imperative to ensure long life on the basis of normal wear of the sliding faces.
A liquid-lubricated seal may have no visible leakage, indicating that the heating and pressure loss in the sealing gap has partly evaporated the fluid film and, thus, the leakage will be in gaseous form or not apparently visible.
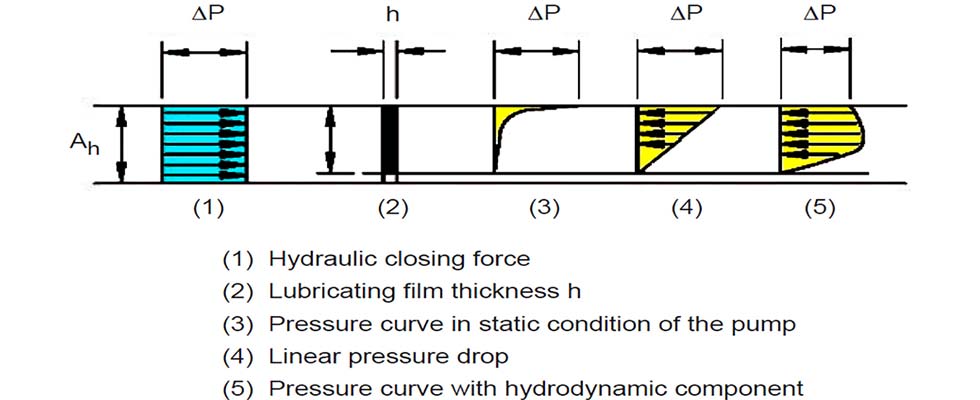 IMAGE 1: Pressure profile in the sealing gap (h) (Image courtesy of Hydraulic Institute)
IMAGE 1: Pressure profile in the sealing gap (h) (Image courtesy of Hydraulic Institute)The leakage rate of a mechanical seal depends on a wide range of factors:
- the roughness and general state of the sliding surfaces during assembly, and over the course of the service life
- flatness of the sliding surfaces and any changes in flatness caused by heat, pressure or mechanically related deformations
- the vibration level and mechanical stability of the pump
- mode of pump operation, i.e., continuous or frequent start/stop
- the fluid characteristics
- Image 1 shows three simplified and idealized pressure profiles in the sealing gap (h), for when the pump shaft is static (3), as the shaft starts to rotate (4), and finally the hydrodynamic zone (5) when the shaft is rotating and the hydrodynamic pressure rises in the gap.

Where:
Q = leakage rate in m3/sec (in3/sec)
r1 = inner face radius in mm (in)
r2 = outer face radius in mm (in)
h = gap height in μm (μin)
ΔP = pressure differential in Pa (psi)
η = dynamic viscosity of the sealed fluid in Pa·s (lbf-sec/in2)
In the hydrodynamic zone, when the opening forces equal the closing forces, the sealing surfaces run a film of liquid, normally without contact and resulting in negligible wear to the seal faces.
Example variables:
r1 = 26.98 mm (1.062 in)
r2 = 31.75 mm (1.25 in)
h = 0.254 μm (total surface roughness) (10 μin)
ΔP = 1.8 MPa (265 psi)
η = 1.0 x 10–3 Pa·s (1.45 x 10–7 lbf-sec/in2)
See Equation 1 for how the theoretical leakage rate of a liquid-lubricated mechanical seal can be calculated.
Using the example variables, the theoretical leakage rate (Q) is 0.34 milliliters per hours (5.86 x 10-6 cubic inches per second).

